Sursă de curent echivalentă
Descriere
Sursă de curent echivalentă
Formula
\(
\begin{aligned}
& I^{\prime}=\frac{U_1}{R_{\mathrm{i} 1}}+\frac{U_2}{R_{\mathrm{i} 2}} \\
& R_{\mathrm{i}}^{\prime}=\frac{R_{\mathrm{i} 1} \cdot R_{\mathrm{i} 2}}{R_{\mathrm{i} 1}+R_{\mathrm{i} 2}} \\
& R_{\text {ers }}=\frac{R_{\mathrm{i}}^{\prime} \cdot R_{\mathrm{L}}}{R_{\mathrm{i}}^{\prime}+R_{\mathrm{L}}}
\end{aligned} \)
Nu ai înțeles ceva? Încearcă cu AI
Science Stories
Explicație
Schema electrică
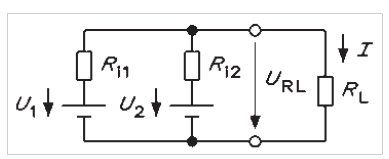
Exemple
I - Curent de scurtcircuit
Ri - Rezistență internă
Rers - Rezistență echivalentă
RL - Rezistență de sarcină
URL - Tensiune la rezistența de sarcină
\( U_{\mathrm{RL}}=I^{\prime} \cdot R_{\text {ers }} \)
\(I=\frac{U_{\mathrm{RL}}}{R_{\mathrm{L}}} \)
Exemplu: U1 = 12 V; U2 = 6 V; Ri1 = 1 Ω; Ri2 = 2 Ω; RL = 10 Ω; I = ?
\( I^{\prime}=\frac{12 \mathrm{~V}}{1 \Omega}+\frac{6 \mathrm{~V}}{2 \Omega}=15 \mathrm{~A} \)
\( R_{\text {ers }}=\frac{0,67 \Omega \cdot 10 \Omega}{0,67 \Omega+10 \Omega}=0,63 \Omega \)
\( U_{\mathrm{RL}}=15 \mathrm{~A} \cdot 0,63 \Omega=9,4 \mathrm{~V} \)
\(R_{\mathrm{i}}^{\prime}=\frac{1 \Omega \cdot 2 \Omega}{1 \Omega+2 \Omega}=0,67 \Omega \)
\(I=\frac{9,4 \mathrm{~V}}{10 \Omega}=0,94 \mathrm{~A} \)
Ri - Rezistență internă
Rers - Rezistență echivalentă
RL - Rezistență de sarcină
URL - Tensiune la rezistența de sarcină
\( U_{\mathrm{RL}}=I^{\prime} \cdot R_{\text {ers }} \)
\(I=\frac{U_{\mathrm{RL}}}{R_{\mathrm{L}}} \)
Exemplu: U1 = 12 V; U2 = 6 V; Ri1 = 1 Ω; Ri2 = 2 Ω; RL = 10 Ω; I = ?
\( I^{\prime}=\frac{12 \mathrm{~V}}{1 \Omega}+\frac{6 \mathrm{~V}}{2 \Omega}=15 \mathrm{~A} \)
\( R_{\text {ers }}=\frac{0,67 \Omega \cdot 10 \Omega}{0,67 \Omega+10 \Omega}=0,63 \Omega \)
\( U_{\mathrm{RL}}=15 \mathrm{~A} \cdot 0,63 \Omega=9,4 \mathrm{~V} \)
\(R_{\mathrm{i}}^{\prime}=\frac{1 \Omega \cdot 2 \Omega}{1 \Omega+2 \Omega}=0,67 \Omega \)
\(I=\frac{9,4 \mathrm{~V}}{10 \Omega}=0,94 \mathrm{~A} \)